Table of Contents:
Chapter 6: The Crumbling of Certainty
Chapter VI: The Crumbling of Certainty
Order without Design
Certain theologians and scientists have made much of the amazing fact that the physical constants of the universe seem to be precisely calibrated to allow the existence of life. The value of the strong nuclear force, the fine structure constant, the gravitational constant, the mass of the electron, and so on would, if different by even a few percent, no longer allow the possibility of life or even, in some cases, stars or solid matter.[8] Some cite this as evidence of a creator setting the stage for life; others invoke a multiplicity of universes, each with different constants. To me the most intriguing is the possibility that the constants actually co-vary with each other and are subject to feedback mechanisms that eventually brought them into the stable attractor configuration they occupy today. In any event, the universe’s pregnancy with order, beauty, and life does not arise from this set of (possibly arbitrary) physical constants alone. Order and beauty are woven into the fabric of reality even more deeply than that. They emerge on every level, in every non-linear system of sufficient complexity. We shall look at a few of these systems at different levels to get some sense of the ubiquity of order—order without design—in the continuing miracle in which we live.
The metaphorical and practical implications of self-organization are staggering, perhaps even more so than those of quantum mechanics. I first became aware of self-organization sixteen years ago when, just out of college, a friend handed me Order out of Chaos by Ilya Prigogine and Isabelle Stengers. This book blew my mind. Amid numerous examples of self-organization in chemical systems, the book gave me my first glimpse of the Mandelbrot Set, an extraordinarily complicated fractal generated by an extremely simple recursive formula. To generate it, take a point “C” in the complex plane and apply the formulas:
Z0=0
Zn+1=Zn2+C
After a given number of iterations, the sequence of Z0, Z1,…, Zn will either exceed absolute value 2, after which it quickly diverges toward infinity, or it will stay in the neighborhood of the origin. If C generates a sequence that stays tame and never diverges toward infinity, it is in the Mandelbrot Set. Unfortunately there is no general, finite way to decide whether that will happen, because even after a billion iterations, it could still start diverging on the billion-and-tenth. In mathematical language, the set is not recursively enumerable, and therefore not decidable either.[9] And this means that there is no shortcut or reason for the set to have the structure it does, no reason except: “That’s the way it is.” The only reason you can (generally) give why a point is in the set is simply to quote the definition: “After N iterations, it still has not diverged.” Our complete reductionistic description of the M. set tells us nothing about it. In effect, here is a reality that cannot be reduced.
We could say the same thing about any random set of points,[10] but what makes the Mandelbrot set special, and a fecund source of metaphor, is that it is evidently not some disorderly scattering of points on the plane, but seems to possess order, structure, and even beauty. Yet a full finite description is impossible; any such description leaves out an infinity of structure. (Notice the parallel with the inescapable reduction of reality entailed in representational language and measure described in Chapter Two.)
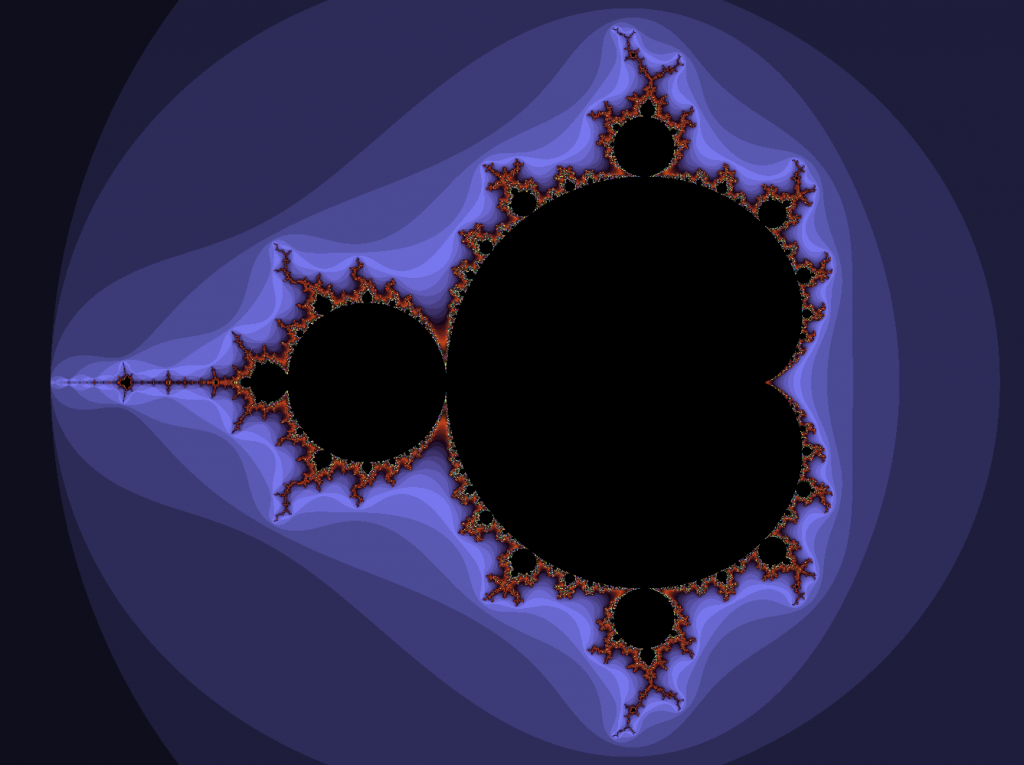
Figure 1: The Mandelbrot Set. The black regions are parts of the set; the colors fade toward blue according to how quickly the points diverge.
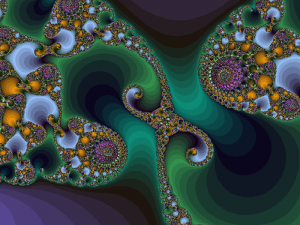
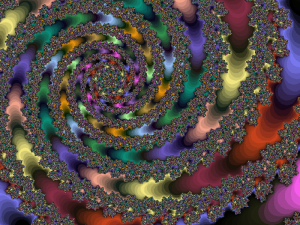
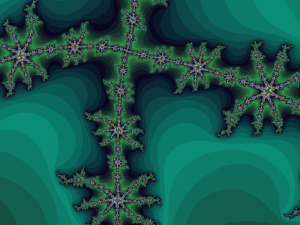
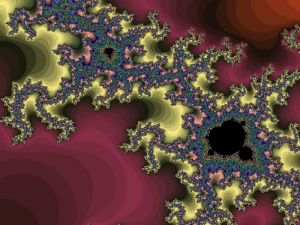
Figure 2: Various parts of the Mandelbrot Set under magnification. Sometimes upon zooming in by several million orders of magnification you can find distorted replicas of the whole set.
The M. set is not unique in the spontaneous emergence of structure—the same thing has been discovered in other mathematical systems such as cellular automata, neural networks, simulations of group behavior, and boolean networks. Stuart Kauffman calls it “order for free”, because it is not evident in the defining equations. Studying boolean networks, Kauffman has even identified general parameter values that virtually ensure order will emerge (even though the only way to find out what that order looks like is to calculate it out).[11]
Let me offer one more example. The cellular automaton called “Langton’s Ant” couldn’t be any simpler. Imagine an ant crawling on a grid where each square must be either black or white. If the ant lands on a black square, he colors it white and turns right. If he lands on a white square, he colors it black and turns left. The ant wanders aimlessly about patterning and repatterning the plane, until at some point (depending on the initial setup) something very strange happens: the ant begins building a “highway” out to infinity.[12] Numerous computer trials confirm that this happens no matter what the initial setup, yet this is an empirical fact only. It is probably impossible to prove it analytically.[13] In other words, the only reason or explanation for “Why does the ant build a highway every time” is “Because it is true for setup A, setup B, setup C, . . .” which is really no explanation at all. Such an explanation amounts to, “Because it does.” As with the M. set, we have a complete reductionistic explanation of the ant’s every move, yet it tells us nothing about the large-scale structure.
In other words, nothing in the simple defining equations of Langton’s Ant would indicate that highway-building behavior will result. There is no finite explanation, yet it happens just the same! There is no simpler “why”. It just is. Neither, therefore, can we be certain that highway-building always happens. We could demonstrate it for a billion starting setups, but it could fail for the billion-and-first. Analogous situations are ubiquitous in the world of cellular automata and related fields, leading researchers such as Stephen Wolfram to advocate a “new kind of science” based on empirical discovery and not analytic proof. Since science is about understanding the world, what they are advocating is really a new conception of understanding, one which is no longer subject to certainty. It becomes necessary to accept that complex systems have emergent properties for which we will never find a reductionistic “why”.
Not only certainty, but also predictability and control fall by the wayside. We must accept that no matter how precise our control over initial conditions, the results might surprise us. With Langton’s Ant we know highway-building will result, but not when or where. Changing merely one square in the initial grid can utterly alter the “when and where”. In chaos theory this is known as “sensitive dependence on initial conditions”, which afflicts not just mathematical systems but physical, chemical, and biological systems as well—anything with non-linearity and feedback . We might empirically infer general information, analogous to the ant’s highway-building, but the details will elude us. For example we can look at earth’s meteorological data and predict that hurricanes will blow through the Caribbean, and explain why, but we are helpless to predict their timing or precise paths. Despite vast improvements in data density, weather forecasting is still inaccurate beyond a few days. Exponential increases in data density bring at best linear improvements in forecast period.
These examples show that determinism and practical predictability are actually two different things. Idealized Newtonian two-body mechanics possesses both: past, present, and future are contained in a single equation, and the evolution of a system at any point in time is easy to calculate. But with few exceptions, this only holds for linear systems. A free pendulum is infinitely predictable; a driven pendulum (where a motor drives the fulcrum back and forth) is chaotic. In a linear system, uncertainties in measurement result in only linear divergence in predicted results—”noise” stays manageably small in comparison to “signal”. But in non-linear systems, the noise quickly overwhelms the signal. A tiny difference in initial conditions results in totally different behavior.
The word “design” implies an intentional awareness of end results that is built in to the initial setup. The engineer desires a certain outcome, and so builds that into his design. Design implies some kind of predictability, properties of the whole that can be predicted from the properties of the parts. When people speak of “intelligent design” as a cosmological theory, this is exactly what they have in mind: an external Creator God who calibrated the initial conditions and laws of the universe in precisely the way that would result in intelligent life. I am suggesting a different conception of God, not as creator but as Creativity itself, not outside the universe but an inseparable property of the universe. Why should the M. set be so ornately beautiful? We hunger for a “why”, but there is none. No reason except, “That’s the way it is.” Reality is just like that. In the Mandelbrot Set I see God, a miraculous mystery that I can never fully grasp, not because of the limits of my mind but because it is constitutionally ungraspable, impervious to reduction. There is nothing to “understand”, nothing to grasp. It is just like that. It is because it is. It is the eternal “I am”. Just as 2+2=4, the M. set could be no other way. Order for free. Beauty for free. Not designed that way, but an irreducible property of reality. I wanted to say “built-in” but even that smuggles in a dualistic reduction of its self-sufficient miracle.
It is ironic indeed that mathematics, which through its “digits” sought to manipulate, tame, and reorder nature, has brought us back to the indubitable realization that reality at its most fundamental level is forever beyond our grasp. And because this is not an oddity of abstract computational mathematics, but broadly true of any non-linear system, the Technological Program of complete control can never come to pass. Time and time again, experience has shown us that however precise our measurement and control, the end results are unpredictable in a non-linear system. The putative successes of the ascent of technology owe themselves to the linearity of machine technology. In linear systems it works: the more precise the control of initial conditions, the more precisely predictable the end result. Our civilization has developed linear technology to its maximum. The more complex a machine becomes, the (exponentially) more relationships among its parts must be considered to keep it “under control”. Control thus comes at an escalating price, a price which, in human terms, is nothing less than the subjugation of life itself to its demands. The exponential conversion of all things to money described in Chapter Four is just one aspect of this. Our present mode of technology only works when its objects are kept simple, linear; when variables are controlled or eliminated. We can control reality only through reducing it: reducing complex ecosystems to the managed forest, the monocultured farm, the suburban lawn; reducing complex chemistries in herbs and food to just so many “active ingredients” and vitamins; reducing the complexity of human social relationships to the orderliness of a planned society.
Modern technological society is an example of a system possessing order (and even hints of beauty) without design. It is a brutal fact that all human attempts to design society from the top down, as opposed to letting it grow organically, have been abject failures. (Witness the present contrast between North Korea and Cuba, both experiencing considerable isolation from the rest of the world. The former—a centralized command economy—is in dire straights while the latter, though poor, gets by with an adequate quality of life thanks to the resilience springing from the high degree of local autonomy there.)[14] The complexity and scale of technological society defies human attempts at design. Any new technological invention, any new law or social innovation, has effects that ripple through the system in unpredictable ways.
In a non-linear system such as a society or an ecology, the effects of any technological fix are wholly unpredictable. Introduce a new species to control a pest, and the end result might be the emergence of an even worse pest. Elevate seratonin levels with a reuptake inhibitor, and the end result might be a reduction in the number of receptors for seratonin and other neurotransmitters, resulting in depression even worse than before. Build a new road to ease congestion, and new developments might end up making congestion all the worse. Cut down all the slow-growing trees and replace them with a fast-growing lumber crop, and diseases might sweep through this weakened ecosystem that result eventually in less lumber, not more. Introduce computers to reduce the drudgery of office work, and people end up spending more time at their desks than ever. Add nitrogen fertilizer to increase soil fertility, and the resulting changes in soil ecology end up reducing its fertility. All of these unpredictable and even contradictory results arise from non-linearity. We can pretend the world is linear and enforce our pretense to a certain extent, but only at increasing cost to freedom and life, because outside a very narrow realm, the world is not in fact linear. Our civilization is built on that tiny segment that is linear, or that can reasonably be viewed that way. Today, that pretense is crumbling. The price has become unbearable. Yet unbearable though it may be, we will continue to bear it as long as we are ignorant of any alternative. We will continue to remedy the failures of control with even more control, intensifying the plundering of earth, life, beauty, and health until all are utterly consumed. That is why it is so important to be aware of another way, suggested in part by the scientific developments I describe in this chapter.
We are inclined to see the unintended consequences of technology as the result of lack of foresight, and to hope that better social engineering might solve the problem. In fact, the problem is insoluble. It is insoluble, that is, by the types of solutions we call “design”. Unable to conceive of other types of solutions, we desperately try harder until we succumb to despair. What other types of solutions might there be that are not designed? How about those that are allowed to grow? The technology flowing from that paradigm might be so different from what we have today as to be unrecognizable.
Someday soon, when we fully digest the futility of control arising from the non-linearity of our universe, we will begin to adopt a wholly different approach to technology, one that does not attempt nature’s reduction but seeks rather its fulfillment. This is not simply a decision about the “appropriateness” of such-and-such a technology; it is the recognition that we are incompetent to determine its ultimate effects. Technological development will come from a place of humility.
The Technological Program of complete control follows from the Scientific Program of complete understanding, in the Newtonian sense of reductionism and predictability. A new technology that is not of control depends therefore on a new mode of understanding. To understand something need not mean to have taken its measure, to know its inner workings, to reduce it analytically. We have now seen the limits of this mode of understanding in physics and mathematics. The next two sections will reveal identical limits in biology and ecology. Some things, perhaps most things, can be understood only as wholes and through their interaction with the rest of the world. Such understanding comes through relationship and experience, even, we could say, through intimacy. No longer can we expect science to make us “lords and possessors of nature”; instead we will see its purpose as being to bring us into more intimate relationship to nature. The spirit of discovery, curiosity, and wonder that is the deepest motivation for science will remain intact.
In the absence of certainty, reason will lose its primacy as the royal road to truth and resume its rightful place as one of several modes of knowledge, each suited to its proper domain. Returning to the alchemical model of Chapter Three, it is the function of the head to reflect, of the heart to know, and of the viscera to transform. The first is cool, the second warm, the third hot. The head—cool reason, cold hard science—has its place; the problem today is that it has usurped the functions of the others. We have built a civilization in which we look to reason, to science, to specialists of all stripes to guide our society and create our future. We have given them a task for which they are constitutionally incapable. Analysis and reason cannot know. They can explore, break apart, reflect, but it is not theirs to know or to choose. They cannot apprehend all the qualities listed in Chapter Three—spirit, beauty, life, consciousness, meaning, purpose, love—that “when you take it apart, are not there.” As a result, all of them are notoriously absent from “cold, hard” science, which, following Galileo’s lead, concerns itself only with the measurable—that which can be reduced to number. Another way of putting it is that science and reason are blind. Without the heart’s guidance their methods are equally capable of good and of evil. Remember that the Holocaust was conducted according to a terrible logic with the utmost rational efficiency. According to a particular choice of first principles, the whole thing was perfectly reasonable. Similarly, enormous scientific and engineering efforts today go toward the manufacture of armaments, harmful chemicals, trashy consumer goods, and all the other instruments of planetary despoliation and cultural impoverishment—again, all in accordance with an implacable economic logic.
Reason cannot evaluate truth. Reason cannot apprehend beauty. Reason knows nothing of love. Living from the head brings us to the same place, whether as individuals or as a society. It brings us to a multiplicity of crises. The head tries to manage them through more of the same methods of control, and the crises eventually intensify. Eventually they become unmanageable and the illusion of control becomes transparent; the head surrenders and the heart can take over once again. We are now very close to this point.
Reason can know nothing of beauty, truth, and love, but it can be put into their service. Reductionistic techniques cannot apprehend the emergent phenomena of wholes, but they can be tools for exploring them. Can you imagine a science dedicated to discovering humanity’s role and function in that vast cosmic Whole of which we are a part? Can you imagine a technology dedicated to the fulfillment of that role? Perhaps that is humanity’s destiny: not lordship over the cosmos, nor meaningless life and death in an indifferent purposeless universe, but conscious participation in a cosmic evolutionary process whose grandeur we can only now begin to imagine.
[8] A detailed technical overview of these constants is offered in the classic The Anthropic Cosmological Principle, by Frank Tippler and John Barrow. Their explanation, which is really an anti-explanation, is that these constants have to be what they are because if they weren’t we wouldn’t be here to even ask about them. Most people find their reasoning sound but deeply unsatisfying. Why are you reading this book? Well, obviously everything in your life that brought you to this book had to be that way, else you wouldn’t be reading this book.
[9] I have recently learned that this statement is not proven. If the hyperbolicity conjecture for the M. set is true, then the set is in a certain sense computable. However, that conjecture remains unproven despite enormous efforts to prove it. Strictly speaking, recursive enumerability only applies to countable sets, but everything I say about the M. set also applies to its intersection with the algebraic complex plane.
[10] By random here I mean not recursively enumerable. Philosophers might quibble with the equation of randomness and non-recursive enumerability, so let me note in passing that this is essentially equivalent to the definition of randomness used in algorithmic information theory (AIT). In AIT a set of numbers is random if there is no computer program shorter than the set itself that can generate the set.
[11] Kauffman, Stuart, At Home in the Universe: The Search for the Laws of Self-Organization and Complexity, Oxford University Press, 1995, and The Origins of Order: Self-Organization and Selection in Evolution, Oxford University Press, 1993.
[12] Provided the initial setup contains a finite number of white squares or a finite number of black squares.
[13] Even if such a proof were to be found, there are other emergent properties of cellular automata that are formally undecidable. John Conway’s “Game of Life” is one example. Conway proved that this cellular automaton can be configured into the equivalent of a universal Turing Machine, which implies via the Halting Problem that there is no finite, general way to decide whether a given starting configuration grows without bound.
[14] Pfeiffer, Dale Allen. Drawing Lessons from Experience: The Agricultural Crises in North Korea and Cuba. http://www.fromthewilderness.com/free/ww3/111703_korea_cuba_1_summary.html. Attempts to plan out artificial societies have been uniform failures. The more determined the effort to exercise centralized control, the more dysfunctional the society becomes: Stalinist Russia and present-day North Korea are two excellent examples. On a smaller scale, communities such as the Owenite experiments of the 19th century disintegrate swiftly unless the foundational “plan” is allowed to coevolve with the society it underlies.